This is a recently published[cite]10.5560/ZNB.2012-0189[/cite] (hypothetical) molecule which has such unusual properties that I cannot resist sharing it with you. It is an annulene with 144 all-cis CH groups, being a (very) much larger cousin of (also hypothetical) systems mooted in 2009[cite]10.1021/ja710438j[/cite],[cite]10.1021/jp902176a[/cite].
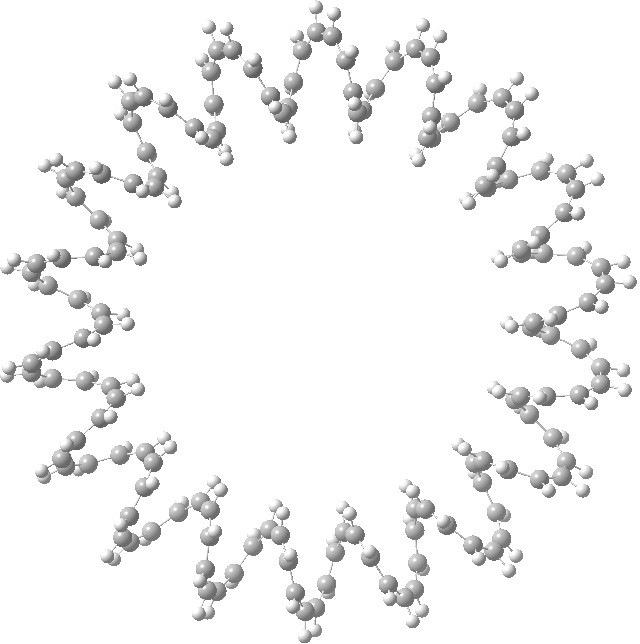
A 144-carbon annulene. Click for 3D.
One fascinating novel aspect of Berger’s work is that he identifies that such helical systems will exhibit a distinct anapolar ring current structure in a constant and homogeneous magnetic field, perpendicular to the main molecular plane. Such anapolar magnetism is distinctly different from the dipolar (diatropic) ring currents normally associated with aromatic molecules, and with the current interest in the magnetic properties of graphene-like objects (see also this blog post and also the helical metal wire) such molecules can only help to excite our imaginations.
I also show one of the more stable molecular orbitals for the [144]-annulene (ωB97XD/6-31G(d,p) calculation).‡ Molecular art indeed!
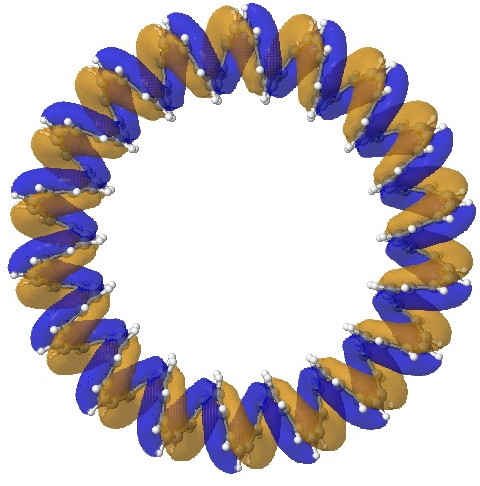
MO 461. Click for 3D.
If you go to the Knotplot site, there you will find a torus link of form (2,18), which displays as the below. Look familiar? Notice the chirality is opposite however!
‡Orbitals for smaller rings with such form can be found here.
Unfortunatly, I missed some previous work on Henry’s related systems when I published that… sad!
But there are some more fascinating aspects I discovered after publication. For instance imagine you expand from ((CH)8)18 – this one- to ((CH)8)n and approach infinity with n. given all of those are minma on the pes you end up with a straight helix. Now this helix has a periodicity of 8 CH units, which would be a structural isomer to the “normal helical polyacetylene” which shows seemingly unrational periodicities between 8 and 9. This is somehow a funny kind of isomerism, though its only a “Gedankenexperiment”.
This may be entirely unconnected, but a few years back, I looked at helical carbon (i.e. no hydrogen atoms). A small unit of cyclic C22 can be made to loop back over itself over a distance of about 8-9 atoms. It thus adopts the shape of a Bernoulli lemniscate (in 3D).
The molecular orbitals of this system are also pretty wacky!
Also not in direct reply, but fascinating: When you lookup “magnetic field in toroidal solenoid” you find that the induced field increases going from the outer to the inner side of the torus-rod. And exactly according to that the chemical shieldings in (CH)144 for 1H and 13C behave like.
Molecular conjugated systems resemble current circuits, but you do not need a power supply, you simply put them into some even static magnetic fields!
There is (I have found out) an extensive literature on helical polyacetylene. One article that caught my eye (there are many) is this one: Two-Dimensional Surface Chirality Control by Solvent-Induced Helicity Inversion of a Helical Polyacetylene on Graphite; DOI: 10.1021/ja061238b. These polymers can be prepared as a single chiral enantiomer (which can be characterised by Electronic circular dichroism), and this article reports a helicity inversion induced by solvent. I find that pretty neat!
I would be interested in an ACID plot of the system. Its something I discovered also only recently, but I think for displaying conjugation its even better suited than the current plots, which are strongly dependent of the relative orientation of mag. field and molecule.
The [144] annulene is a 4n, n=36 electron closed shell system. If ρ( r) is in fact a torus link (even numbered linking number Lk in units of π) then it should not be what would conventionally described as aromatic. In fact, the bond lengths around the ring do not alternate much, which suggests that it is in fact delocalised (and hence by one criterion aromatic). But in truth, I do not suppose that the Hückel 4n+2/4n rules that are normally used in these circumstances have ever been tested for such large annulenes with so many electrons.
It might transpire that such anapolar systems are in a class of their own when it comes to describing their aromaticity! It is not every day that a truly new form of aromaticity is discovered.
So yes, the ACID plot would indeed be interesting. As would eg the properties of a dication or dianion of the [144] annulene.
When I remember correctly, upon adding or subtracting 2 electrons the scf does not converge anymore. The orbital energies are quite dense already (and/or the geometry is not stable anymore).
ps. The lemniscate is incredibly fascinating!
It seems as if Raphael and I are conducting an open science investigation here (something perhaps easier to do on a blog than in a conventional medium?). I gave the dication a go, and the result can be inspected at this digital repository link. It seems that the bond alternation increases, from ~1.39/1.42Å to 1.36/1.44 (ωB97XD/6-31G(d,p) level to allow for dispersion to develop) when two electrons are removed from helical [144]-annulene (in C18 symmetry). It returns to ~1.39/1.42 when four electrons are removed. So two electrons do seem to have a big effect (given that the system has 144 π-like electrons in total).
Would be a hint at a 4n-pi rule? The currents I integrated were quite large, both para and diatropic ones about double of what you have in benzene. But then HOMO and LUMO are a1 and a2, and for an “aromat” wouldnt you expect to have some degenerate HOMO and LUMO?
In Möbius systems, the degeneracies are only approximate, and do not arise from group theory (this arises because of the finite width of the band, and there are only degeneracies if the band is a wire of zero thickness).
There are many planar π-systems which sustain both para and diatropic ring currents, often in a concentric manner, and so it would be no surprise to find both types in a helical system (although the term concentric might have to be modified).
Perhaps a comparison of the ring currents for the neutral 144-electron system with the dicationic 142-electron system might be interesting?
What would you expect for an odd-numbered C-chain, lets say [((CH)9)17]+?
For investigating such systems we would need a good algo for generating starting coordinates for ((CH)n)m. Ideally it would read in n, m and the average C-C length. But then there is at least one more degree of freedom describing how “stretched” the system is. This parameter would couple both radii of the “torus” (lets use this term here for simplicity). Complicated task!
For an odd-numbered chain, the topology changes from a link to a knot. There are an odd number of crossings in the electron density torus. The MOs can no longer be considered singly, but one must add pairs of (approximately degenerate) MOs. In effect, the pseudo-MO now “traverses” the cycle twice (as appropriate for any odd-twisted band).
![A torus knot of order [2,13].](http://www.ch.imperial.ac.uk/rzepa/blog/wp-content/uploads/2013/02/knot-2-13.jpg)
As for generating the coordinates, yes, not easy! When we constructed smaller objects up to say C7 symmetry (ie C35H35+) a brute-force method of sketching out the molecule and letting the geometry optimiser do the initial refinement, followed by attempted symmetrisation managed the trick. But this would not work for these larger systems.
Oh, now I see 🙂
I’ll try to do first the currents in [144]2+.
Rob Scharein, the mathematician who produced Knotplot, clearly has worked out algorithms for doing this sort of thing. You might try to contact him?
A sideways shift! The image below is of the NCI (non-covalent-interactions) for the neutral [144]-annulene. It looks at the topology of the electron density in the non-covalent region, and colour codes it according to the second eigenvalue of the density Hessian. This reveals mildly attractive regions (green) and mildly repulsive regions (yellow). The technique attempts to identify regions were eg mildly attractive dispersion forces might be operating. As you can see, there is a LOT of dispersion in this helix; it is in some ways the ultimate stacked system!
Cool!
Btw. the structure motive itself is realized in nature in cyclic DNA or RNA strands – is it messenger RNA ? It does not need alltoomuch of speculation to assume that there is also lots of dispersion in these.
Regarding the odd-twisted bands and the possibilities for a Möbius-curent: I always get in trouble when trying to understand how a diatropic odd-twist-Möbius-current could be explained in the MO-picture. You might know Patrick Fowler’s series of papers on the MO basis of mag. induced currents (like doi: 10.1351/pac200779060969).
The bottom line is that diatropic currents are the result of virtual transitions with translational symmetry and paratropic ones of virtual transitions of rotational symmetry (each weighted with the difference between the orbital energies), (which in some beautiful way explains the paratropic nature of the currents in cyclobutadiene). My problem now with pi-Orbitals and odd-twist Möbius currents is, that one would need some (set of) totally symmetric MOs from which the virtual excitation starts. And this appears to me somehow impossible since the phases of pi-orbitals cannot be positive and negative at the same time.
Or simply: How can π-conjugation take place over the whole odd-twist-Möbius band?
Regarding the last point, you can start at any node in the p-AO basis of an odd-twist Möbius system and track its overlap continuously until you reach the start. You will have made two circuits of the basis by doing this. If the system has a lot of writhe, the overlap between any two adjacent AOs will be almost optimal (since there is little twist left in the system). If you take a pair of MOs of this type, and square and add them, you get a continuous torus of electron density with no other nodes. That torus looks exactly like the orange torus knot reproduced above. I suppose we can call that conjugation. For an even twist system, you get instead two torus curves for the density, each making only one circuit of the system; this is called a torus link.
Perhaps because you need to do two circuits, that is how you can get both positive and negative overlaps at the same time?
Re: cyclic dna. Indeed yes, the linking number of such dna is often analysed in terms of the sum of the twist and the writhe. Here to express whether the duplex is over-coiled or under-coiled. It is of course the two individual chains of dna that forms the helix. With π-systems, the two nodes of each p-AO play the role of the dna chains.
As a helical system, the [144] annulene would be expected to have an optical rotation. Using the modern version of the theory introduced by Kirkwood (see here), and using ωB97XD/6-31G(d,p), [α]589 and [α]880 can be obtained as -209088° and +73952° respectively (for the P-helical form). In other words the ORD (optical rotatory dispersion) is enormously varying with wavelength.
I suspect there are many sources of error for this calculation, but nevertheless, these numbers are startlingly large!
I have (re)optimized the [((CH)8)18]2+ (how to get the indices here?) at B-P/SV(P) level in D18, without dispersion correction (for consistency with my previous calculations). I find bond lengths almost equal in size between 1.399 and 1.401 Angströms (LES frequency analysis is running). Then I found a spectacularly large magnetically induced ring current: in toal 49 nA/T (57 diatropic and -8 nA/T paratropic, thereof). I dare say that is world record for a pure hydrocarbon. For comparison in ((CH)8)18 its 15 nA/T in total (30 diatropic and -15 nA paratropic). My results hint at an extremely strong aromaticity for 4n+2. At the moment I have no explanation for the disagreement with Henry’s results from the ωB97XD/6-31G(d,p) calculation. Can it be only dispersion? I continue checking my results.
The only other explanation is a different electron occupancy? Or that one or the other is not a stable minimum in D18 symmetry. I too am doing a frequency calculation for the 2+ system, and we can compare results. It might also be that single-ζ basis sets over-symmetrize? Thus hydrogen bonds in such a basis are often placed in a single-well rather than a double-well potential?
Normally, if one changes an electron count by 2 in such a system, the diatropic/paratropic ring currents invert (see for example 10.1021/ol703129z) so this system is unusual no matter how it emerges!
If the bond length result of 1.399/1.401 for the bonds in the di-cation is the true answer, then that also would be intriguing. Shaik has argued that the distortivity of π-electrons means that in planar annulenes, the bonds should start to become unequal/alternate at about 26 electrons or greater. Here we would be seeing no such effect at 142 electrons! Perhaps the helical twist has attenuated the distortivity?
Orbital occ. is ok. 1006 electrons. The 14 highest occ. orbitals are of e type, lumo is a1. total energy is -5567.87560 in (CH)8)18 it is 5568.230915. For the ((CH)8)18 most results from SV(P) were very close to TZVP ones, I cannot remember principal differences. freq still running …
btw. isn’t sv(p) double zeta?
The neutral system is actually _very_ paramagnetic with 15 nA/T paratropic current (something I probably could not gauge in the original work, because the 30 nA diatropic currents are also huge). In total it is of course diamangnetic since its closed shell, like rectangular cyclobutadiene, which is in total also diamagnetic but shows large paratropic currents and is an anti-aromat like ((CH)8)18).
It seems the 4n+2 and 4n rules are fullfilled, however the current in the dication is huge and the shieldings in ((CH8)18)2+ are also extreme. I will report these values soon.
On this last point then, if we could keep the 144 electrons (=4n) but switch the topology of the electron density from a torus link to a torus knot (by adding one extra C(+) atom say) then we should get a huge diatropic rather an paratropic current!
Yes, I would expect that. Btw. Rob is interested! I am awaiting exteremly excited coordinates for [(((CH)9)17)]-, or would you expect any troubles from an anion? I would go for 9-fold periodicity since the “normal polyacetylenes” as I mentioned above are between 8 and 9, and 7 might be too much strain.
Sorry, I have missunderstoos your last question. At the moment I do not fully understand the influence of the topology on the direction of the currents. All I see is that 4n+2 is aromatic and 4n is anti-aromatic. So I simply would go for the odd-twist-Möbius system and give it 4n+2 electrons.
I think 4n is anti-aromatic for even (zero) twists, and aromatic for odd twists. Conversely, 4n+2 is aromatic for even (zero) twists and anti-aromatic for odd twists. So I think the interesting one would be a 4n electron system with odd twists.
I agree, 9 is better than 7, but I think the difference may mostly impact upon the dispersion interactions. Also, anions need better basis sets than cations!
The logic is, that you have two separate loops for the link and one twice as large loop for the knot, right? Wouldn’t we then need 2n+1 electrons for the knot??
All the thermal Möbius systems so far characterised are 4n for odd twists and 4n+2 for even (non-zero) twists. See e.g. 10.1021/ol703129z for two examples.
The factor of two you note above relates to the basis functions, not the electron count. So I still think that for eg 144 electrons, a system with an odd twist (actually, formally one does not call it a twist, one calls it a linking number, since the twist is just one component of the linking number, the other being the writhe) is the interesting one.
I see. So we take [((CH)9)17)]+?
Because the [144] annulene is helical, it should have a prominent electronic circular dichroism spectrum. Here is the prediction (for the (P) helix), ωB97XD/6-31G(d,p) calculation (first 15 electronic states only). The Cotton effect (Δε) is indeed huge (it rarely reaches 500 for most measured molecules). The excitation wavelength (~800 nm) corresponds to an electronic excitation approaching the far infra-red.
Maybe we have to divide all properties by ten for reasons of fairness (it has 288 atoms!) 😉
You might want to do this, but famously, another helical system, DNA, has very small Cotton effects in its ECD spectrum!
The BP86/TZVP/D18 of [144]2+ is cose to convergence. The short C-C are about 1.398 and the long ones about 1.4040. With SV(P) it was 1.4078 1.4132. The LES has still not converged.
These are systems with unusual correlation, and hence highly dependent on eg the nature of the DFT treatment. Thus with B3LYP/6-311G(d,p) I get: neutral [144]-annulene 1.44/1.36Å; (doi: 10.6084/m9.figshare.155843) and 2+ [144]-annulene 1.42/1.38Å (doi: 10.6084/m9.figshare.155846). The 4n+2 system clearly alternates less than the 4n system, and so the standard Hückel rule still applies!
The classic test of bond alternation is in fact [26]annulene, which is not helical but flat. I gave a talk at the ACS on this topic, and one slide summarises the results I obtained for this smaller annulene. Its importance is that some (e.g Sason Shaik) think it is the annulene that represents the onset of bond alternation, with all larger annulenes also alternating (it is the region where π-distortivity finally wins out over σ-symmetrisation, is per benzene, and as I describe here). I compared eg the B2GP-B2PLYP/TZVPP method (a double hybrid method in which short range correlation is handled by DFT theory and long range by MP2 theory) with eg B3LYP, and found they were more or less similar (i.e. [26] annulene shows a small amount of bond alternation, [22] none at all, and [30] a fair bit).
So I think one good test would be to repeat this using B86 for [26] annulene. Perhaps in the zoo of functionals, B86 is at one extreme of fully delocalising the bonds (Hartree Fock methods are at the other of localising them).
Ultimately, we would like to test these against eg CCSD(T), but this is currently not possible.
OK, I tried the combination B97D/6-311G(d,p) (Gaussian does not support a simple B86 functional), and found 1.398/1.398Å for [26] annulene (doi: 10.6084/m9.figshare.155859).
So the issue perhaps boils down to which functional is the most appropriate for studying bond-length alternation in larger annulenes (and whether any particular method scales well as you increase the size). I suspect that any one method may apply well to perhaps just a short range of scales.
For what it is worth, I did try another calibration for a [38] nonaphyrin, for which an X-ray is known. This is a 4n+2 annulene with an even (double) twist which as an aromatic molecule might be expected to show little bond alternation, and it is also helical! The experimental difference in the meso bond lengths was measured as Δ0.014Å. RHF gets Δ0.115, and B3LYP is Δ0.012Å. So, what does B86 (or B97D) get for this calibrant, perhaps the closest we currently have as a model for the [144]-annulene?
Could you please provide with the coordinates of [22]-Annulene? Then I’ll give it a shot.
Sorry i meant [26]!
The repository links (using B3LYP) are in the above comment! If you want to try the [30] annulene it is here.
I have tracked down two decaphyrins which have helical structures not that different from the [144] annulene. The Cambridge codes are SELQUW and HIYTAL. The latter had bond alternation at a meso position of ~ 1.39/1.43. doi: 10.1002/chem.200600158 and 10.1002/chem.200701909.
RI-BP86/SV(P)/D2h for [26]:
dist 3 c — 11 c = 2.6542 au = 140.45 pm
dist 5 c — 6 c = 2.6542 au = 140.45 pm
dist 16 c — 18 c = 2.6542 au = 140.45 pm
dist 22 c — 23 c = 2.6542 au = 140.45 pm
dist 8 c — 9 c = 2.6545 au = 140.47 pm
dist 10 c — 12 c = 2.6545 au = 140.47 pm
dist 15 c — 17 c = 2.6545 au = 140.47 pm
dist 25 c — 26 c = 2.6545 au = 140.47 pm
dist 1 c — 2 c = 2.6549 au = 140.49 pm
dist 1 c — 4 c = 2.6549 au = 140.49 pm
dist 19 c — 20 c = 2.6549 au = 140.49 pm
dist 20 c — 21 c = 2.6549 au = 140.49 pm
dist 7 c — 8 c = 2.6570 au = 140.60 pm
dist 12 c — 13 c = 2.6570 au = 140.60 pm
dist 14 c — 15 c = 2.6570 au = 140.60 pm
dist 24 c — 25 c = 2.6570 au = 140.60 pm
dist 6 c — 7 c = 2.6573 au = 140.62 pm
dist 11 c — 14 c = 2.6573 au = 140.62 pm
dist 13 c — 16 c = 2.6573 au = 140.62 pm
dist 23 c — 24 c = 2.6573 au = 140.62 pm
dist 9 c — 10 c = 2.6768 au = 141.65 pm
dist 17 c — 26 c = 2.6768 au = 141.65 pm
dist 2 c — 3 c = 2.6773 au = 141.68 pm
dist 4 c — 5 c = 2.6773 au = 141.68 pm
dist 18 c — 19 c = 2.6773 au = 141.68 pm
dist 21 c — 22 c = 2.6773 au = 141.68 pm
Yes, pretty much the same as B97D (is that a more recent version of B86?). I am giving B97D a go with that nonaphyrin I mentioned in an earlier comment. At least it is an x-ray structure that the calculations can be compared with. And perhaps those helical decaphyrins.
Its actually abbreviated “b-p” in TM:
func | type |exch | corr.
b-p | GGA | S+B88 | VWN(V)+P86
but b97d is implemented as well:
b97-d | GGA | B97 refit | B97 refit
What code is “TM”? It’s not listed in this compilation.
Turbomole.
Because DFT methods are not properly variational, it can be really quite tricky to directly compare the results from two different implementations of the same method. I gather for example that B3LYP as implemented in Gaussian is not quite the same as B3LYP implemented in other codes. If a Turbomole result for eg B97D for [26] can be compared with that using Gaussian as quoted above, that would calibrate the degree of difference due to the two different codes.
The D18 structure of [144]2+ in BP86/SV(P) has no imaginary frequencies. The C-C distances range from 1.405 to 1.417 Å
The B-P97D/SV(P) distances without dispersion corr. on are:
dist 3 c — 11 c = 2.6543 au = 140.46 pm
dist 5 c — 6 c = 2.6543 au = 140.46 pm
dist 16 c — 18 c = 2.6543 au = 140.46 pm
dist 22 c — 23 c = 2.6543 au = 140.46 pm
dist 8 c — 9 c = 2.6548 au = 140.49 pm
dist 10 c — 12 c = 2.6548 au = 140.49 pm
dist 15 c — 17 c = 2.6548 au = 140.49 pm
dist 25 c — 26 c = 2.6548 au = 140.49 pm
dist 1 c — 2 c = 2.6551 au = 140.50 pm
dist 1 c — 4 c = 2.6551 au = 140.50 pm
dist 19 c — 20 c = 2.6551 au = 140.50 pm
dist 20 c — 21 c = 2.6551 au = 140.50 pm
dist 7 c — 8 c = 2.6575 au = 140.63 pm
dist 12 c — 13 c = 2.6575 au = 140.63 pm
dist 14 c — 15 c = 2.6575 au = 140.63 pm
dist 24 c — 25 c = 2.6575 au = 140.63 pm
dist 6 c — 7 c = 2.6577 au = 140.64 pm
dist 11 c — 14 c = 2.6577 au = 140.64 pm
dist 13 c — 16 c = 2.6577 au = 140.64 pm
dist 23 c — 24 c = 2.6577 au = 140.64 pm
dist 9 c — 10 c = 2.6775 au = 141.69 pm
dist 17 c — 26 c = 2.6775 au = 141.69 pm
dist 2 c — 3 c = 2.6779 au = 141.71 pm
dist 4 c — 5 c = 2.6779 au = 141.71 pm
dist 18 c — 19 c = 2.6779 au = 141.71 pm
dist 21 c — 22 c = 2.6779 au = 141.71 pm
The one with dispersion on follow.
btw there is also a “Gaussian” type b3lyp in Turbomole, the Gaussian version apparently uses the VWN(III) while Turbomole uses VWN(V). Seems that there are some different versions of the Vosko-Wilk-Nussair correlation functional around.
Here the b97-d with dispersion on:
dist 3 c — 11 c = 2.6530 au = 140.39 pm
dist 5 c — 6 c = 2.6530 au = 140.39 pm
dist 16 c — 18 c = 2.6530 au = 140.39 pm
dist 22 c — 23 c = 2.6530 au = 140.39 pm
dist 1 c — 2 c = 2.6535 au = 140.41 pm
dist 1 c — 4 c = 2.6535 au = 140.41 pm
dist 8 c — 9 c = 2.6534 au = 140.41 pm
dist 10 c — 12 c = 2.6534 au = 140.41 pm
dist 15 c — 17 c = 2.6534 au = 140.41 pm
dist 19 c — 20 c = 2.6535 au = 140.41 pm
dist 20 c — 21 c = 2.6535 au = 140.41 pm
dist 25 c — 26 c = 2.6534 au = 140.41 pm
dist 7 c — 8 c = 2.6554 au = 140.52 pm
dist 12 c — 13 c = 2.6554 au = 140.52 pm
dist 14 c — 15 c = 2.6554 au = 140.52 pm
dist 24 c — 25 c = 2.6554 au = 140.52 pm
dist 6 c — 7 c = 2.6558 au = 140.54 pm
dist 11 c — 14 c = 2.6558 au = 140.54 pm
dist 13 c — 16 c = 2.6558 au = 140.54 pm
dist 23 c — 24 c = 2.6558 au = 140.54 pm
dist 9 c — 10 c = 2.6759 au = 141.60 pm
dist 17 c — 26 c = 2.6759 au = 141.60 pm
dist 2 c — 3 c = 2.6765 au = 141.63 pm
dist 4 c — 5 c = 2.6765 au = 141.63 pm
dist 18 c — 19 c = 2.6765 au = 141.63 pm
dist 21 c — 22 c = 2.6765 au = 141.63 pm
I mentioned earlier try another calibration for a [38] nonaphyrin, for which an X-ray is known. The experimental difference in the meso bond lengths was measured as Δ0.014Å. RHF gets Δ0.115, and B3LYP is Δ0.012Å.
To this I can now add a B97D/6-311G(d,p) calculation (doi: m9.figshare.156033) giving 0.007 as the bond alternation (averaged over 6 meso-positions). This is about half the experimental (x-ray) value. So up to about 38 electrons, I think this method (and B86?) are under-estimating the degree of bond alternation. Molecule HIYTAL, a [46] electron system, is rather bigger, and at the limit of what might be calculated, but its worth a go I think. After that, we might tentatively be in a position to extrapolate to 144 electrons! (?).
Molecule SELQUW I have noticed is a [56] dodecaphyrin which is actually smaller than the [46] decaphyrin HIYTAL. Both have helical winds related to the [144] annulene that started this discussion, and both are viable calculation candidates (but it will take a few days to optimise their geometries at the levels discussed here). So watch this space.
[…] extensive discussion developed regarding my post on a fascinating helical [144]-annulene. Topics included the nature of the ring current sustained […]
[…] first attempt was to 3D print a molecular orbital; in fact the one shown in this post. To be printable, a 3D object must be fully connected, in other words not contain any disconnected […]