Henry Rzepa's Blog

This is a continuation of the previous post exploring the transition state geometries of various types of ring closure as predicted by  Baldwin’s rules. I had dealt with bond formation to a trigonal (sp²) carbon; now I add a digonal (sp) example (see an interesting literature variation). 

As before, I have added two solvent (water) molecules to the model, since the immediate product of the closure is a zwitterionic intermediate, which is likely to be stabilised by the solvent. I also used the same nucleophile as before to facilitate comparison.

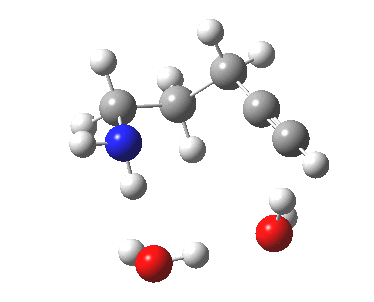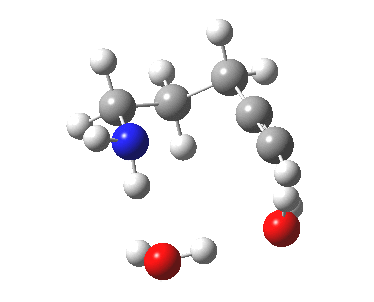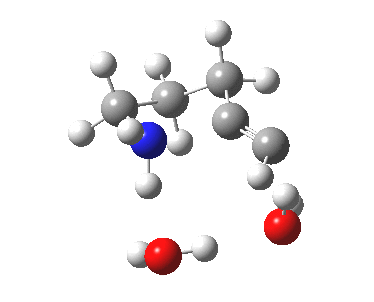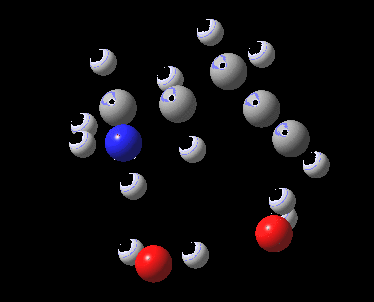
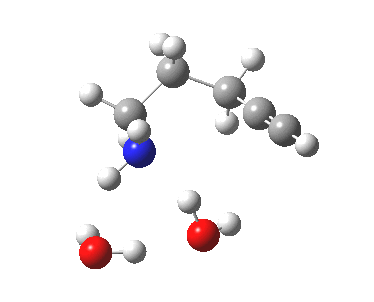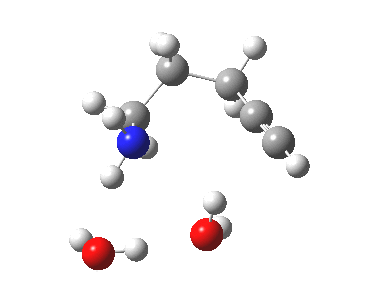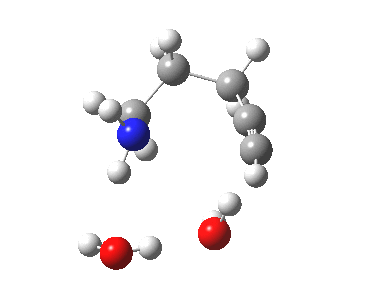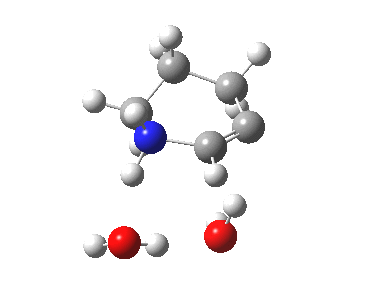
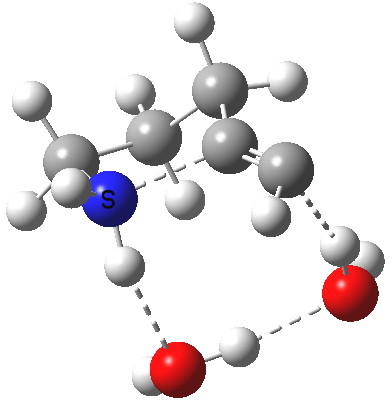5-exo-dig transition state. Click for 4D.

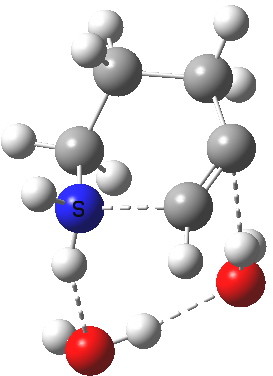6-endo-dig transition state. Click for 4D.

The digonal angle of attack is 121° for the  exo form, and 116° for the endo, both larger than was the case in the trig systems. The relative free energies of the two transition states is 3.6 kcal/mol in favour of the exo isomer. The hydrogen bond network is somewhat strained, since two solvent molecules cannot quite reach the forming carbanion at the optimal angle to form a good hydrogen bond to it. Instead, the water has to content itself with a π-facial hydrogen bond between the alkyne and the H-O. As a result, proton transfer to the carbon requires a separate activation step (or a stronger acid than water). 

5-exo-dig transition state

6-endo-dig transition state

The IRC for the 6-endo-dig pathway has features worth commenting upon.

1. At IRC -12, the two solvent molecules form a triangular network with the nucleophilic amine.
2. By IRC -9, one of the water molecules has split itself off from this triangle, and started to move towards the triple bond, which is gradually becoming a better acceptor of a hydrogen bond.
3. At IRC -3, this water molecule is now forming a  π-facial hydrogen bond to the alkyne, which is still largely in place at the end of this step of the mechanism.

To complete the mechanism, I have added the final step in the reaction, a proton transfer from the amine to the carbon recipient, as facilitated by the bridge of solvent molecules connecting the start and end of the process. The free energy of this transition state is 0.3 kcal/mol higher than the N-C bond forming reaction, making it (just) the rate determining step.

Proton transfer
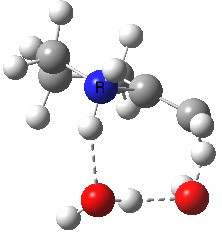Transition state for proton transfer. Click for 4D

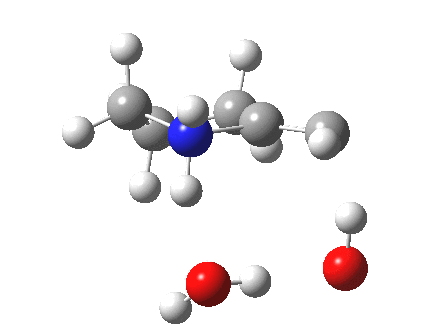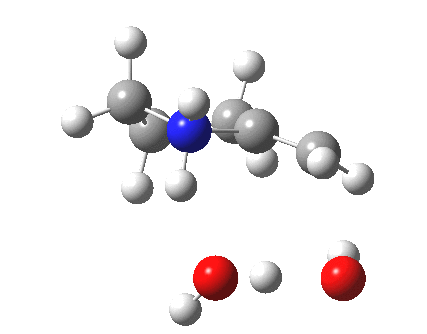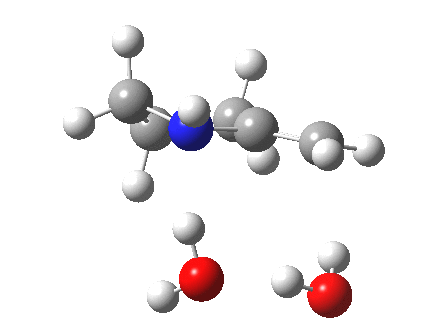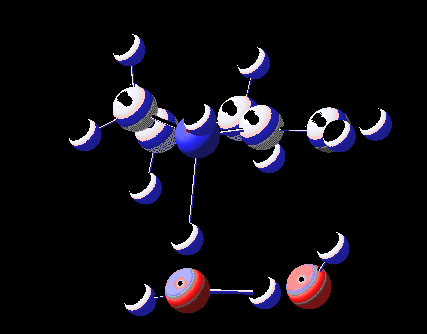1. The feature at IRC = 0.0 (the transition state) is the first proton transfer, from  C to O.
2. The second feature at  IRC -2.5 is an O to O proton transfer
3. At IRC -4, the third and final proton transfer can be seen, from O to N.
4. At IRC -6.5, a weak π-OH hydrogen bond forms.

There is one more common type of cyclisation covered by Baldwin’s rules, this time involving tet(rahedral) or sp³ centres. This turns out to be the most interesting of the lot; reporting on this will have to wait a little!

Tags: Baldwins rules, free energy, hydrogen bond network, immediate product, Reaction Mechanism

This entry was posted on Sunday, June 10th, 2012 at 6:33 pm and is filed under Uncategorised. You can follow any responses to this entry through the RSS 2.0 feed. You can leave a response, or trackback from your own site.